LU decomposition of a matrix with complete pivoting, and related features. More...

#include <FullPivLU.h>

Public Types
enum	{ RowsAtCompileTime = MatrixType::RowsAtCompileTime, ColsAtCompileTime = MatrixType::ColsAtCompileTime, Options = MatrixType::Options, MaxRowsAtCompileTime = MatrixType::MaxRowsAtCompileTime, MaxColsAtCompileTime = MatrixType::MaxColsAtCompileTime }
typedef MatrixType::Index	Index
typedef internal::plain_col_type < MatrixType, Index >::type	IntColVectorType
typedef internal::plain_row_type < MatrixType, Index >::type	IntRowVectorType
typedef _MatrixType	MatrixType
typedef PermutationMatrix < RowsAtCompileTime, MaxRowsAtCompileTime >	PermutationPType
typedef PermutationMatrix < ColsAtCompileTime, MaxColsAtCompileTime >	PermutationQType
typedef NumTraits< typename MatrixType::Scalar >::Real	RealScalar
typedef MatrixType::Scalar	Scalar
typedef internal::traits < MatrixType >::StorageKind	StorageKind
Public Member Functions
Index	cols () const
FullPivLU &	compute (const MatrixType &matrix)
internal::traits< MatrixType > ::Scalar	determinant () const
Index	dimensionOfKernel () const
	FullPivLU ()
	Default Constructor.
	FullPivLU (Index rows, Index cols)
	Default Constructor with memory preallocation.
	FullPivLU (const MatrixType &matrix)
const internal::image_retval < FullPivLU >	image (const MatrixType &originalMatrix) const
const internal::solve_retval < FullPivLU, typename MatrixType::IdentityReturnType >	inverse () const
bool	isInjective () const
bool	isInvertible () const
bool	isSurjective () const
const internal::kernel_retval < FullPivLU >	kernel () const
const MatrixType &	matrixLU () const
RealScalar	maxPivot () const
Index	nonzeroPivots () const
const PermutationPType &	permutationP () const
const PermutationQType &	permutationQ () const
Index	rank () const
MatrixType	reconstructedMatrix () const
Index	rows () const
FullPivLU &	setThreshold (const RealScalar &threshold)
FullPivLU &	setThreshold (Default_t)
template<typename Rhs >
const internal::solve_retval < FullPivLU, Rhs >	solve (const MatrixBase< Rhs > &b) const
RealScalar	threshold () const
Static Protected Member Functions
static void	check_template_parameters ()
Protected Attributes
IntRowVectorType	m_colsTranspositions
Index	m_det_pq
bool	m_isInitialized
MatrixType	m_lu
RealScalar	m_maxpivot
Index	m_nonzero_pivots
PermutationPType	m_p
RealScalar	m_prescribedThreshold
PermutationQType	m_q
IntColVectorType	m_rowsTranspositions
bool	m_usePrescribedThreshold

Detailed Description

template<typename _MatrixType>
class Eigen::FullPivLU< _MatrixType >

LU decomposition of a matrix with complete pivoting, and related features.

Parameters:

MatrixType the type of the matrix of which we are computing the LU decomposition

This class represents a LU decomposition of any matrix, with complete pivoting: the matrix A is decomposed as 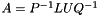$A = P^{-1} L U Q^{-1}$ where L is unit-lower-triangular, U is upper-triangular, and P and Q are permutation matrices. This is a rank-revealing LU decomposition. The eigenvalues (diagonal coefficients) of U are sorted in such a way that any zeros are at the end.

This decomposition provides the generic approach to solving systems of linear equations, computing the rank, invertibility, inverse, kernel, and determinant.

This LU decomposition is very stable and well tested with large matrices. However there are use cases where the SVD decomposition is inherently more stable and/or flexible. For example, when computing the kernel of a matrix, working with the SVD allows to select the smallest singular values of the matrix, something that the LU decomposition doesn't see.

The data of the LU decomposition can be directly accessed through the methods matrixLU(), permutationP(), permutationQ().

As an exemple, here is how the original matrix can be retrieved:

Output:

See also:: MatrixBase::fullPivLu(), MatrixBase::determinant(), MatrixBase::inverse()

Definition at line 46 of file FullPivLU.h.

Member Typedef Documentation

template<typename _MatrixType>

typedef MatrixType::Index Eigen::FullPivLU< _MatrixType >::Index

Definition at line 60 of file FullPivLU.h.

template<typename _MatrixType>

typedef internal::plain_col_type<MatrixType, Index>::type Eigen::FullPivLU< _MatrixType >::IntColVectorType

Reimplemented in Eigen::LU< MatrixType >.

Definition at line 62 of file FullPivLU.h.

template<typename _MatrixType>

typedef internal::plain_row_type<MatrixType, Index>::type Eigen::FullPivLU< _MatrixType >::IntRowVectorType

Reimplemented in Eigen::LU< MatrixType >.

Definition at line 61 of file FullPivLU.h.

template<typename _MatrixType>

typedef _MatrixType Eigen::FullPivLU< _MatrixType >::MatrixType

Definition at line 49 of file FullPivLU.h.

template<typename _MatrixType>

typedef PermutationMatrix<RowsAtCompileTime, MaxRowsAtCompileTime> Eigen::FullPivLU< _MatrixType >::PermutationPType

Definition at line 64 of file FullPivLU.h.

template<typename _MatrixType>

typedef PermutationMatrix<ColsAtCompileTime, MaxColsAtCompileTime> Eigen::FullPivLU< _MatrixType >::PermutationQType

Definition at line 63 of file FullPivLU.h.

template<typename _MatrixType>

typedef NumTraits<typename MatrixType::Scalar>::Real Eigen::FullPivLU< _MatrixType >::RealScalar

Reimplemented in Eigen::LU< MatrixType >.

Definition at line 58 of file FullPivLU.h.

template<typename _MatrixType>

typedef MatrixType::Scalar Eigen::FullPivLU< _MatrixType >::Scalar

Reimplemented in Eigen::LU< MatrixType >.

Definition at line 57 of file FullPivLU.h.

template<typename _MatrixType>

typedef internal::traits<MatrixType>::StorageKind Eigen::FullPivLU< _MatrixType >::StorageKind

Definition at line 59 of file FullPivLU.h.

Member Enumeration Documentation

template<typename _MatrixType>

anonymous enum

Enumerator:

RowsAtCompileTime
ColsAtCompileTime
Options
MaxRowsAtCompileTime
MaxColsAtCompileTime

Definition at line 50 of file FullPivLU.h.

Constructor & Destructor Documentation

template<typename MatrixType >

Eigen::FullPivLU< MatrixType >::FullPivLU ( )

Default Constructor.

The default constructor is useful in cases in which the user intends to perform decompositions via LU::compute(const MatrixType&).

Definition at line 394 of file FullPivLU.h.

template<typename MatrixType >

Eigen::FullPivLU< MatrixType >::FullPivLU	(	Index	rows,
		Index	cols
	)

Default Constructor with memory preallocation.

Like the default constructor but with preallocation of the internal data according to the specified problem size.

See also:: FullPivLU()

Definition at line 400 of file FullPivLU.h.

template<typename MatrixType >

Eigen::FullPivLU< MatrixType >::FullPivLU ( const MatrixType & matrix )

Constructor.

Parameters:

matrix the matrix of which to compute the LU decomposition. It is required to be nonzero.

Definition at line 412 of file FullPivLU.h.

Member Function Documentation

template<typename _MatrixType>

static void Eigen::FullPivLU< _MatrixType >::check_template_parameters ( ) [inline, static, protected]

Definition at line 378 of file FullPivLU.h.

template<typename _MatrixType>

Index Eigen::FullPivLU< _MatrixType >::cols ( void ) const [inline]

Definition at line 374 of file FullPivLU.h.

template<typename MatrixType >

FullPivLU< MatrixType > & Eigen::FullPivLU< MatrixType >::compute ( const MatrixType & matrix )

Computes the LU decomposition of the given matrix.

Parameters:

matrix the matrix of which to compute the LU decomposition. It is required to be nonzero.

Returns:: a reference to *this

Definition at line 425 of file FullPivLU.h.

template<typename MatrixType >

internal::traits< MatrixType >::Scalar Eigen::FullPivLU< MatrixType >::determinant ( ) const

Returns:: the determinant of the matrix of which *this is the LU decomposition. It has only linear complexity (that is, O(n) where n is the dimension of the square matrix) as the LU decomposition has already been computed.

Note:: This is only for square matrices.; For fixed-size matrices of size up to 4, MatrixBase::determinant() offers optimized paths.

Warning:: a determinant can be very big or small, so for matrices of large enough dimension, there is a risk of overflow/underflow.

See also:: MatrixBase::determinant()

Definition at line 515 of file FullPivLU.h.

template<typename _MatrixType>

Index Eigen::FullPivLU< _MatrixType >::dimensionOfKernel ( ) const [inline]

Returns:: the dimension of the kernel of the matrix of which *this is the LU decomposition.

Note:: This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Definition at line 312 of file FullPivLU.h.

template<typename _MatrixType>

const internal::image_retval<FullPivLU> Eigen::FullPivLU< _MatrixType >::image ( const MatrixType & originalMatrix ) const [inline]

Returns:: the image of the matrix, also called its column-space. The columns of the returned matrix will form a basis of the kernel.

Parameters:

originalMatrix the original matrix, of which *this is the LU decomposition. The reason why it is needed to pass it here, is that this allows a large optimization, as otherwise this method would need to reconstruct it from the LU decomposition.

Note:: If the image has dimension zero, then the returned matrix is a column-vector filled with zeros.; This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Example:

Output:

See also:: kernel()

Definition at line 188 of file FullPivLU.h.

template<typename _MatrixType>

const internal::solve_retval<FullPivLU,typename MatrixType::IdentityReturnType> Eigen::FullPivLU< _MatrixType >::inverse ( void ) const [inline]

Returns:: the inverse of the matrix of which *this is the LU decomposition.

Note:: If this matrix is not invertible, the returned matrix has undefined coefficients. Use isInvertible() to first determine whether this matrix is invertible.

See also:: MatrixBase::inverse()

Definition at line 363 of file FullPivLU.h.

template<typename _MatrixType>

bool Eigen::FullPivLU< _MatrixType >::isInjective ( ) const [inline]

Returns:: true if the matrix of which *this is the LU decomposition represents an injective linear map, i.e. has trivial kernel; false otherwise.

Note:: This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Definition at line 325 of file FullPivLU.h.

template<typename _MatrixType>

bool Eigen::FullPivLU< _MatrixType >::isInvertible ( ) const [inline]

Returns:: true if the matrix of which *this is the LU decomposition is invertible.

Note:: This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Definition at line 350 of file FullPivLU.h.

template<typename _MatrixType>

bool Eigen::FullPivLU< _MatrixType >::isSurjective ( ) const [inline]

Returns:: true if the matrix of which *this is the LU decomposition represents a surjective linear map; false otherwise.

Note:: This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Definition at line 338 of file FullPivLU.h.

template<typename _MatrixType>

const internal::kernel_retval<FullPivLU> Eigen::FullPivLU< _MatrixType >::kernel ( ) const [inline]

Returns:: the kernel of the matrix, also called its null-space. The columns of the returned matrix will form a basis of the kernel.

Note:: If the kernel has dimension zero, then the returned matrix is a column-vector filled with zeros.; This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Example:

Output:

See also:: image()

Definition at line 162 of file FullPivLU.h.

template<typename _MatrixType>

const MatrixType& Eigen::FullPivLU< _MatrixType >::matrixLU ( ) const [inline]

Returns:: the LU decomposition matrix: the upper-triangular part is U, the unit-lower-triangular part is L (at least for square matrices; in the non-square case, special care is needed, see the documentation of class FullPivLU).

See also:: matrixL(), matrixU()

Definition at line 104 of file FullPivLU.h.

template<typename _MatrixType>

RealScalar Eigen::FullPivLU< _MatrixType >::maxPivot ( ) const [inline]

Returns:: the absolute value of the biggest pivot, i.e. the biggest diagonal coefficient of U.

Definition at line 126 of file FullPivLU.h.

template<typename _MatrixType>

Index Eigen::FullPivLU< _MatrixType >::nonzeroPivots ( ) const [inline]

Returns:: the number of nonzero pivots in the LU decomposition. Here nonzero is meant in the exact sense, not in a fuzzy sense. So that notion isn't really intrinsically interesting, but it is still useful when implementing algorithms.

See also:: rank()

Definition at line 117 of file FullPivLU.h.

template<typename _MatrixType>

const PermutationPType& Eigen::FullPivLU< _MatrixType >::permutationP ( ) const [inline]

Returns:: the permutation matrix P

See also:: permutationQ()

Definition at line 132 of file FullPivLU.h.

template<typename _MatrixType>

const PermutationQType& Eigen::FullPivLU< _MatrixType >::permutationQ ( ) const [inline]

Returns:: the permutation matrix Q

See also:: permutationP()

Definition at line 142 of file FullPivLU.h.

template<typename _MatrixType>

Index Eigen::FullPivLU< _MatrixType >::rank ( ) const [inline]

Returns:: the rank of the matrix of which *this is the LU decomposition.

Note:: This method has to determine which pivots should be considered nonzero. For that, it uses the threshold value that you can control by calling setThreshold(const RealScalar&).

Definition at line 295 of file FullPivLU.h.

template<typename MatrixType >

MatrixType Eigen::FullPivLU< MatrixType >::reconstructedMatrix ( ) const

Returns:: the matrix represented by the decomposition, i.e., it returns the product: 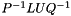$P^{-1} L U Q^{-1}$ . This function is provided for debug purposes.

Definition at line 526 of file FullPivLU.h.

template<typename _MatrixType>

Index Eigen::FullPivLU< _MatrixType >::rows ( void ) const [inline]

Definition at line 373 of file FullPivLU.h.

template<typename _MatrixType>

FullPivLU& Eigen::FullPivLU< _MatrixType >::setThreshold ( const RealScalar & threshold ) [inline]

Allows to prescribe a threshold to be used by certain methods, such as rank(), who need to determine when pivots are to be considered nonzero. This is not used for the LU decomposition itself.

When it needs to get the threshold value, Eigen calls threshold(). By default, this uses a formula to automatically determine a reasonable threshold. Once you have called the present method setThreshold(const RealScalar&), your value is used instead.

Parameters:

threshold The new value to use as the threshold.

A pivot will be considered nonzero if its absolute value is strictly greater than 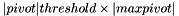$\vert pivot \vert \leqslant threshold \times \vert maxpivot \vert$ where maxpivot is the biggest pivot.

If you want to come back to the default behavior, call setThreshold(Default_t)

Definition at line 255 of file FullPivLU.h.

template<typename _MatrixType>

FullPivLU& Eigen::FullPivLU< _MatrixType >::setThreshold ( Default_t ) [inline]

Allows to come back to the default behavior, letting Eigen use its default formula for determining the threshold.

You should pass the special object Eigen::Default as parameter here.

 lu.setThreshold(Eigen::Default);

See the documentation of setThreshold(const RealScalar&).

Definition at line 270 of file FullPivLU.h.

template<typename _MatrixType>

template<typename Rhs >

const internal::solve_retval<FullPivLU, Rhs> Eigen::FullPivLU< _MatrixType >::solve ( const MatrixBase< Rhs > & b ) const [inline]

Returns:: a solution x to the equation Ax=b, where A is the matrix of which *this is the LU decomposition.

Parameters:

b	the right-hand-side of the equation to solve. Can be a vector or a matrix, the only requirement in order for the equation to make sense is that b.rows()==A.rows(), where A is the matrix of which *this is the LU decomposition.